All published articles of this journal are available on ScienceDirect.
Reviewing Construction Schedule Float Management
Abstract
As the complexity in construction projects increased, simply managing the obtained float values and critical path(s) by the CPM method usually results in more difficult schedule control and, consequently, in incorrect decision making due to non-realistic float values. This study thoroughly reviewed various float types in the literature and professional project management systems, and discussed five managerial essentials and three proactive strategies on mitigating challenging float-related problems based on the perspective of managing schedules by controlling floats. With some comments and suggestions, the outcomes of this study not only improve the knowledge level on schedule management but also provide a better understanding of float management to improve the quality of schedule management.
INTRODUCTION
The critical path method (CPM) is a technique that the construction industry has broadly implemented in managing project schedules since it was first developed in the 1950s. With the analytical results of the CPM, project managers usually manage projects by emphasizing two management measures: critical path(s)/activities and float variations. Obviously, schedule delay problems appear in almost all construction projects, although many professional project management systems (PMSs) have provided complicated and flexible schedule management functionalities based on the fundamentals of CPM. For managing project schedules, the critical path(s)/activities and floats are the key measures usually used to reflect the management focus and flexibility of activities. When the complexity of construction projects increased, these two measures require considerable attention in managing projects proactively.
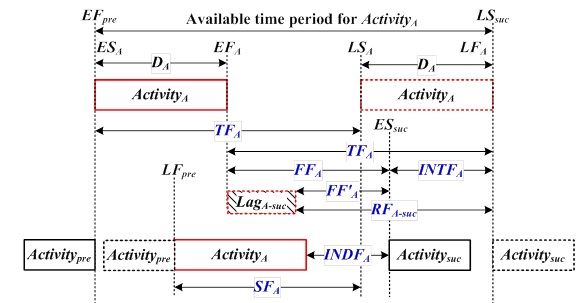
Float, defined as the time the performance of an activity may be delayed without delaying overall project completion [1], is a resource that provides flexibility in the contractor’s operation [2]. In project planning, each activity has its float values based on a CPM calculation. Furthermore, when a project is delayed or proceeds ahead of the planned schedule, recalculating its schedule to obtain updated float values for remaining activities is necessary. Within a traditional CPM calculation, four types of float can be calculated: total float (TF), free float (FF), interfering float (INTF) and independent float (INDF). Fig. (1) shows a graphical representation for the popular float types, which are discussed in the next section.
For helping the CPM calculation, two graphical approaches, i.e. the arrow diagramming method (ADM) and the precedence diagramming method (PDM), were developed. Initially, commercial professional PMSs generated ADM networks for use in the construction industry; however, in the past several years, most of the popular professional PMSs did not provide ADM networks in their updated versions. Considering the practice perspective, this study merely discusses the floats in PDM.
TF and FF are the primary float types used in the industry, whereas INTF and INDF are only used marginally [3]. From the management’s perspective, the various types of float are important measures of schedule flexibility and its ability to absorb future changes and deviations [4]. Therefore, the purpose of this study is to review comprehensively all floats defined in different sources to understand and use them better in managing construction projects. Based on the perspective of managing schedules by controlling floats and the experience of the author on teaching, consultant and dispute resolution on schedule management in Taiwan, this study discussed five managerial essentials and three proactive strategies on mitigating challenging float-related problems to provide a chance of employing available float types. Although the discussed managerial essentials and proactive strategies are limited to the viewpoint of this study, the outcomes of this study could be a start point for better schedule management if schedule manager and project manager entirely understand the definitions and functions of floats in PMSs.
The remainder of this paper is organized as follows: the next section reviews the literature on float definitions to provide a basis for further discussion. The third section reviews the floats developed and employed in advanced scheduling approaches, i.e., linear scheduling, resource-constrained scheduling and critical chain scheduling, to examine whether the floats in advanced scheduling approaches bring innovative methods for float management. The fourth section reviews the floats used in professional PMSs, i.e., Microsoft® Office Project, Oracle® Primavera® P6TM and Deltek® Open PlanTM, to examine whether the PMSs provide easy-to-use float management functions. The fifth section discusses five managerial essentials for managing project floats and the sixth section discusses three unsolved issues and proposes accompanying proactive strategies for mitigating the challenging float-related problems. Finally, conclusions are drawn in the last section, along with recommendations for future research.
REVIEW OF FLOAT DEFINITIONS
Total Float
Total float (TF) is a by-product of the CPM calculation [5]; TF is the difference between the time available for performing an activity and the time required for doing it [1]. TF is the least restrictive definition of float [1]; however, TF is the most popular float type in managing construction projects. Based on project management perspective, TF is defined as the total amount of time that a scheduled activity may be delayed from its early start date without delaying the project finish date, or violating a schedule constraint [6]. TF represents a meaning of total and maximum float for an activity. Fig. (1) shows the time available for ActivityA considering its predecessor (Activitypre) and successor (Activitysuc), the duration of ActivityA (DA), and the temporal slot for the TF of Activity A (TFA). Thus, TF is calculated using the CPM technique and determining the difference between the early finish dates and the late finish dates [6]. Furthermore, TF is defined as the time span in which the completion of an activity may occur and not delay the termination of the project [7]. That is, TF is the maximum time an activity can be delayed without delaying project completion [8]. Based on a simplified logical relationship (finish-to-start), the calculation of TF can be formulated as Equation (1), in which ES is the early start time, EF is the early finish time, LS is the late start time and LF is the late finish time. Furthermore, in Equation (1), the time difference between ActivityA and its successor (ActivitySuc) is denoted as LagA-suc (time lag).
For complicated logical relationships (start-to-start, finish-to-finish and start-to-finish), TF can be transformed into start total float (STF), representing the difference between LS and ES of an activity and, alternatively, into a finish total float (FTF), representing the difference between LF and LS.
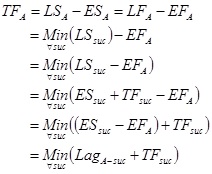 |
(1) |
The value of TF belonging to critical activities in a schedule network may be positive, negative, or zero. Where project completion is concerned, activities with float times can be delayed without extending the time [9]. In general, the activity on the critical path (the longest path) in a network has a TF value of zero. A negative TF value implies that the targeted project completion date is earlier than the project completion date calculated by the CPM. In contrast, if the targeted project completion date is later than the project completion date, the TF have a positive value for the critical activities. Therefore, the critical activities are those with the smallest total float values on the longest path in a network, whether the TF values are positive, negative, or zero.
Notably, TF is the amount of float available to an entire chain or path of activities; TF indicates the flexibility of all activities on the same path. Therefore, TF is shared among these same-path activities; it does not necessarily represent the float available to a specific or singular activity.
Free Float
FF is defined as the time span in which the completion of an activity may occur and not delay the finish of the project nor delay the start of any following activity [7]. That is, FF is the amount of time that a schedule activity can be delayed without delaying the early start date of any immediately following schedule activities [6]. Thus, FF is the maximum time an activity can be delayed without delaying the start of any succeeding activity [8]. In Fig. (1), the time span of FFA is clearly displayed. Based on the simple finish-to-start relationship, the calculation of FF can be formulated as Equation (2); thus, FF can be illustrated as the minimum time period between ActivityA and its successor (Activitysuc). The maximum value of FF will be equal to TF. Unlike TF, FF is the property of an activity and is not shared with any other activities in the schedule network [9].
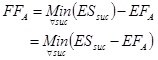 |
(2) |
Normally, no time lag is added between two activities. However, in complicated logical relationships, the time lag between two activities will be arranged and influence the value of FF. For example, if a positive time lag (LagA-suc) is added to the relationship between activity A and its successor, the value of FF is decreased. Equation (3) shows that time lag (LagA-suc) is considered for calculating FF (FF'A). Thus, the apparent value of FF can be manipulated using time lag. Therefore, the activity requiring physical time lag should be managed carefully while considering the variations of FF.
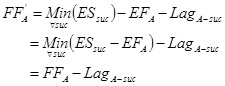 |
(3) |
Interfering Float
INTF is defined as the time span in which the completion of an activity may occur and not delay the termination of the project but within which the completion will delay the start of some other following activity [7]. Based on the simple finish-to-start relationship, the calculation of INTF can be formulated by Equation (4), in which, the value of INTF is equal to the value of TF minus FF for an activity. Thus, TF can be divided into two parts: FF and INTF. If an activity has a positive TF, FF and INTF, delays on this activity will consume first its FF and then INTF.
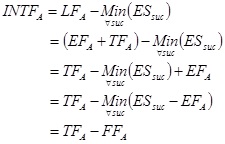 |
(4) |
In Fig. (1), the time span for INTFA is displayed to represent the difference between TFA and FFA. Clearly, for , if the successor (Activitysuc) is started at its late start, it is not influenced by ActivityA.
Independent Float
INDF is defined as the time span in which the completion of an activity may occur and not delay the termination of the project, not delay the start of any following activity, and not be delay by any preceding activity [7]. Thus, INDF is the amount of delay that can be assigned to any activity without delaying subsequent activities or restricting the scheduling of preceding activities [8]. In the calculation perspective, INDF is calculated as the minimum of all ESs of successors minus the duration of the current activity minus the maximum of all LFs of predecessors. Equation (5) shows the INDF calculation formula, in which DA is the duration of activity A.
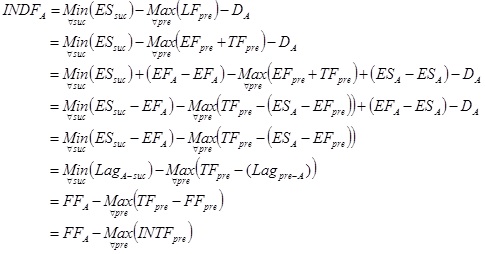 |
(5) |
The time span for in Fig. (1) shows that the value might be less than. From Equation (5), it is also clear that INDFA. can be obtained by FFA minus the predecessors’ maximum interference (INTFpre) which is usually greater or equal to zero. The purpose of calculating INDF is to isolate where float can be used on one activity and is not available to any other activity [1]. INDF predicts the worst case scenario where “pressure” is exerted onto an activity from both sides (predecessor and successor) [10].
Safety Float
For measuring how much the current activity can be delayed without impacting the project finish if all its predecessors are delayed, the safety float (SF) is defined to represent the difference between the LS of the current activity and the maximum of all LFs of predecessors [11-13]. Fig. (1) shows the spatial time span, and Equation (6) shows the calculation formulation for SFA.
 |
(6) |
Based on Equation (6), it is clear that the SF of an activity is the leeway for scheduling all of its predecessors without affecting itself [14]. That is, using SF, a project manager can focus on other activities, excluding the activities with positive SF values.
Relationship Float
Relationship float (RF) is defined as a logical constraint between two activities to represent the criticality of a logical relationship, calculated through the first line of Equation (7) [15]. RF can be transformed into another formula represented in other lines of Equation (7). Namely, RF for an activity is the time buffer to consider when individually allowing for sufficient time lags for its successors. One activity might have multiple RFs.
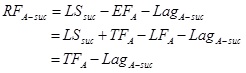 |
(7) |
RF can be further divided into relationship total float (RTF) and relationship free float (RFF) [16]; these floats can be calculated by WST® Open PlanTM (now merged into Deltek®). RFL is defined as the time amount by which the lag on that relationship would have to be increased to delay the successor activity; RTF is defined as the time amount by which the lag would have to be increased to cause a delay in the completion of the overall project (or the violation of a late target) [17].
As-Built Float
As-Built float (ABF) is a time estimate of the activity’s float at the time when the work actually occurred. If the project’s status were noted and every day entered into a project schedule and the CPM were then recomputed, then the ABF would be the daily TF reading for any activity that was ongoing or that could logically start but had not [18]. ABF can be a measure to reflect the updated TF for performing pending activities.
ABF is completely different than TF or FF. Current scheduling software is not designed to calculate or display ABF. Because the CPM is a dynamic measurement of the interactions of activities as unequal progress occurs and other events (planned or unplanned) transpire, this measured value may actually change from one time period to the next, even within the same activity [18].
REVIEW OF FLOATS IN ADVANCED SCHEDULING APPROACHES
In the construction industry, some advanced scheduling approaches have been developed to solve complicated project schedule problems. Although this study only discussed the floats used in three major approaches, i.e., linear scheduling, resource-constrained scheduling and critical chain scheduling, certain floats used in other advanced scheduling approaches in the literature were also summarized for reference.
Floats in Linear Scheduling
Scheduling activities of linear and repetitive projects, the linear scheduling method [19], with various names (line of balance, construction planning technique, vertical production method, time-location matrix model, time space scheduling method, disturbance scheduling, horizontal and vertical logic scheduling for multistory projects, time versus distance diagrams, linear balance charts, and velocity diagrams) being annotated in one previous study [20], attempts to optimize construction productivity and resource continuity. The scheduling problems encountered by linear and repetitive projects can be regarded as special instances of conventional construction projects. The similar concept of floats was broadly used in linear scheduling approaches.
As discussed in linear scheduling methods, the location and time buffers (time floats) were defined and used in indicating how close activities can become while being conducted [13, 21]. The rate float, representing the amount that the production rate of a non-controlling linear activity can be lowered before the activity will become a controlling segment, is used to evaluate the impact of a delay on the project completion [22]. The time float, representing the amount of time a particular activity can be delayed without affecting the scheduled project date, is used to calculate the difference between either the starts or the finishes of an activity and its direct successor [23]. Based on the float definitions of the traditional CPM, a previous study defined potential floats, including TF, FF, INTF, INDF and SF, for linear schedules by using singularity functions to provide a tool to determine how linear or repetitive construction projects were impacted by delays [13].
Above linear scheduling methods adopted the traditional CPM as a basis for developing innovative approaches to solve the particular problems in linear and repetitive projects. Therefore, the floats used in the traditional CPM can be used in linear scheduling approaches. That is, no obvious difference exists between the floats used in the traditional CPM and linear scheduling approaches.
Floats in Resource-constrained Scheduling
The resource-constrained scheduling method is another scheduling technique to minimize project duration, whereas the resource-leveling technique targets to level/smooth resource requirement profiles. Compared to the traditional CPM that assumes unlimited resources, the resource-constrained scheduling method attempts to arrange activities while considering resource availability and limitations.
With considering resource conflict, a previous study defined current float to represent the finish float available with respect to the float’s latest finish time in original network computations. The current float can be regarded as an abstract concept and may take negative values to indicate that the activities are already behind schedule [24]. Furthermore, in considering the resource availability, scheduled total float and scheduled free float were defined to replace original TF and FF in the traditional CPM [4]. In solving implementation problems in resource-constrained scheduling, a previous study identified the problem of the difference between the theoretically remaining TF and the real remaining TF, termed as the phantom float, and proposed an innovative resource-constrained critical path method (RCPM) technique to identify the resource dependency and to keep track of the activity’s late finish time by considering both technological relationships and resource dependencies. Similar to the basic concept of TF shared for activities on the same chain in the traditional CPM, the RCPM technique had an approach to determining which activities (might not be in the same chain) share total floats [25].
Resource-constrained scheduling methods focused on solving resource-constrained problems that the traditional CPM encountered. That is, resource-constrained scheduling methods extend the CPM’s ability in more complicated situations. Similar to the situation of floats in linear scheduling approaches, resource-constrained scheduling methods used not only the floats developed for the traditional CPM but also employed evolution floats to represent specified activity flexibility.
Floats in Critical Chain Scheduling
The critical chain scheduling (CCS) technique derived from the Theory of Constraints (TOC) in the project management domain is an innovative technique to scheduling activities by considering both logic relationships and resource availability [26] and removing some management pitfalls (for example, student syndrome). The CCS technique proposed a new leveled critical path (termed critical chain) instead of the traditional critical path to handle critical activities [27].
Similar to the concept of float in the traditional CPM, many types of buffer were defined: project buffer, feeding buffer and resource buffer [27, 28]. Buffers are designed quantities of time for a project schedule to protect what is important to project success. For improving the robustness of project baseline schedules under CCS, the strategy of choosing appropriate buffer sizes is important and discussed elsewhere [29].
The use of buffers in CCS differs from the use of floats in the traditional CPM due to their fundamental purposes: the former one is used for proactive project management and the latter one is used for reactive project management. However, some commercialized CCS software packages, for example the ProChain® Project Scheduling software [30], integrated the functions of CCS and CPM and provided the float values for activity control and the buffer values for project management. Thus, there is no conflict between the use of floats and the use of buffers in CCS for the same project.
Floats in Other Advanced Scheduling Approaches
In developing an innovative scheduling method for construction projects, the chronographic method, six new types of float were proposed: complete float, start float, finish float, partial complete float, partial start float and partial finish float [3]. Combined with the four popular floats in traditional CPM, the chronographic method derived twenty-four floats, although most of them are theoretically based outcomes, to help schedule an optimized project schedule. The newly developed floats can be regarded as the subclass of traditional CPM floats [3].
For solving several problems (for example, if the level of duration detail is rough) in the traditional CPM, the critical path segments (CPS) approach was developed for better schedule analysis. Based on the CPS approach, the total float in the traditional CPM can be calculated accurately for better schedule management, although the developed approach was not mature [31].
Summary of Floats in Advanced Scheduling Approaches
The floats in three major approaches (linear scheduling, resource-constrained scheduling and critical chain scheduling) have been reviewed for capturing constructive information for better schedule management. Notably, excluding the popular floats in the traditional CPM, the floats in specific advanced scheduling approach are only suitable for the project types specified in particular approach.
In sum, the innovative floats in advanced scheduling approaches are derived from the popular floats in the traditional CPM. Thus, no completely new float was proposed in the discussed scheduling approaches. While using the advanced scheduling approaches, understanding all floats in the traditional CPM is necessary.
Review of Floats in Professional Project Management Systems
Based on practical implementation perspective, manually calculating all float values for a construction project is tedious, time-consuming and even impossible. Commercial PMSs are usually employed to obtain required float values. There are many diversified professional PMSs available now, and many web sites for software review and comparison are also reachable. This study discussed the definition and functionality of floats in the latest version of three popular systems for construction projects: Microsoft® Office Project, Oracle® Primavera® P6TM and Deltek® Open PlanTM, which were selected and discussed in a textbook introducing the CPM for construction management [32]. Basic information of floats in discussed PMSs is summarized in Table 1.
| Software | Used Float Types | Definition for TF | Definition for FF | Key Difference Compared to Other PMSs |
|---|---|---|---|---|
| Microsoft® Office Project | TF, FF, start float and finish float | The amount of time an activity’s finish date can be delayed without delaying the project’s finish date | The amount of time that an activity can be delayed without delaying any successor activity | It defines the negative total float to display the amount of negative total float for an activity on the Gantt Chart |
| Oracle® Primavera® P6TM | TF, FF, start float and finish float | The amount of time the selected activity can be delayed without delaying the project’s finish date | The amount of time the selected activity can be delayed without delaying the immediate successor activities | It defines the float path to representing the path made of activities that drive the activity with the least TF/FF |
| Deltek® Open PlanTM | TF, FF, finish total float, finish free float, relationship total float and relationship free float | The difference between the early and late start dates measured in working periods of the activity calendar |
The maximum amount by which an activity can be delayed beyond its early dates without delaying any successor activity beyond its early dates |
It defines the scheduled float to the difference between the late finish date and the scheduled finish date of the activity |
Floats in Microsoft® Project
The Microsoft® Project name for “float” is “slack.” As noted in Microsoft® Project’s reference file, the behavior of TF can differ depending on the link type. For example, if a user works with a start-to-start relationship, TF can be changed when the user enters progress on the predecessor activity. With progress entered in the predecessor, the start date of the successor is known and becomes part of the total float calculation [33].
Excluding the TF and FF, Microsoft® Project defines start and finish floats for better schedule control. The smaller of the start float and finish float amounts determines the amount of free float available, that is, the amount of time an activity can be delayed without affecting the start date of a successor activity or the project finish date. Notably, if an activity has an actual start date and a deadline, the start float is zero and the finish float is the difference between the activity’s finish date and deadline date [33].
Note that Microsoft® Project 2010 provides a field, the negative slack (negative total float), to display the amount of negative total float for an activity on the Gantt Chart. The negative float indicates that there is not enough time scheduled for the activity and is usually caused by constraint dates or activity dependencies; therefore, the field indicates the amount of time that must be saved so that successor activities are not delayed [33].
Floats in Oracle® Primavera® P6TM
Primavera® P6TM uses TF, FF, start float and finish float for representing the time buffer for delay activity/project. For providing a consistent value for TF, it allows the user to select one of three options: (1) start float; (2) finish float; and (3) the smallest of start float and finish float [34]. The TF might be different to others’ when complicated logical relationships are used.
In determining critical activities, Primavera® P6TM allows the user to define activities either on the longest path or with total float less than or equal to a customized time. Moreover, Primavera® P6TM provides a new function of float path for the user to manage the most critical path (and sub-critical float paths) in a project schedule by considering TF/FF values. Notably, in calculating multiple critical float paths, critical activities that are not part of a critical float path remain tagged as critical [34].
Floats in Deltek® Open PlanTM
Based on a general float definition, the number of time periods by which an activity can be delayed without affecting other activities in a project, Deltek® Open PlanTM, uses TF, FF, finish TF, finish FF, relationship TF and relationship FF for flexible schedule management. Notably, it defines that the relationship TF is the amount by which the lag in a relationship would have to be increased to either cause a delay in the project completion or a late target date; the relationship FF is the amount by which the lag in a relationship would have to be increased to cause the delay of a successor activity [35]. Therefore, the schedule manager can use the relationship TF/FF to determine which relationship is responsible for controlling the critical status of the successor activity.
Note that Open PlanTM calculates a scheduled float for an activity when resource scheduling is executed. The scheduled float is the difference between the late finish date and the scheduled finish date of the activity. In some circumstances, scheduled float may be shared with other activities in the logical flow of the project [35].
Summary of Floats in Commercial PMSs
Through reviewing all float information in three major commercial PMSs (Microsoft® Office Project, Oracle® Primavera® P6TM and Deltek® Open PlanTM), this study finds that only TF and FF in the traditional CPM are common used in discussed PMSs. The definitions of TF and FF summarized in Table 1 are similar. Furthermore, all PMSs drive start and finish TF/FF for facilitating logical relationships of start-to-start and finish-to-finish used. It is interesting that every PMS develops its own unique attribute/function as Table 1 shown for better schedule management.
In sum, all PMSs provide easy-to-use float management functions. Notably, compared to Microsoft® Project and Primavera® P6TM, Open PlanTM provides more complicated float values for the user to control complex project schedules. However, Open PlanTM increases use complexity and might cause wrong use, and even decision making faults if the user employs complicated floats but does not understand them correctly.
Float Management Essentials
Based on the perspective of managing schedules by controlling floats, this study discussed five managerial essentials those are lessons learned from the literature review in this study and the author’s experience.
Representing Flawless Float Values
The scheduler, however, can manipulate diverse float values by using artificial lead/lags, unprecedentedly long activity durations, preferential logic relationships and other constraints. Furthermore, some informal schedule development approaches, for example, using multiple calendars [36], lead to unreasonable and un-predictable float values. When managing float in a construction project, the project manager/scheduler needs to decipher all assumptions and options of schedule developments; otherwise, he/she might manage the project based on unrealistic/unreasonable float values.
As concluded by a previous study, a professional project scheduler should have discipline (scheduling knowledge), experience and honesty; otherwise, he/she ends up with a schedule that is at best useless and at worst deceptive [37]. Disclosing flawless float values based on a generally recognized scheduling practice for all project stakeholders and sharing potential risks and benefits from float management improve the possibility of project success.
Managing Correct Floats in PMSs
Using PMSs to plan and control project schedules is a must for the project manager and scheduler. According to available PMSs, when advanced logical relationships (i.e., start-to-start, finish-to-finish and start-to-finish relationships) and multiple calendars are used, the scheduler should display suitable float types (i.e., start and finish floats) as well as early and late bar charts for identifying key managerial activities, driving relationships and complicated float values to manage project schedules. Otherwise, hidden floats (the float value is hidden and does not appear in TF or FF) might appear in a partially critical activity, i.e., only the activity’s start or finish time is critical. For example, an activity has a five-day finish TF but a zero-day start TF when the start-to-start and finish-to-finish logical relationships are used concurrently. This makes PMSs determining the activity critical. However, this study identifies the activity having five-day hidden float.
Furthermore, float values would be changed while a project is in-progress. Regularly updating project schedules and monitoring the variations of floats for coming activities can easily identify possible schedule delays. Some PMSs have provided “remaining float” for monitoring float values. Notably, different PMSs provide different processes or steps to update a project schedule. For example, the timing, i.e., start or end of a day, for updating the schedule is different for various PMSs. The scheduler should manage critical activities with the start and finish floats simultaneously; otherwise, managing a critical activity merely based on the criterion of critical/non-critical might lead to wrong decision making.
Maintaining Positive Floats
Positive floats reflect that the activity with these float values has a large completion buffer time. However, practical experience has shown that nearly independent work in a project is relatively rare; thus, a high free float value often identifies missing logical relationships. When the project manager and scheduler want to manage floats proactively, they should understand float definitions clearly because float values for the project and activity levels have different connotations.
For a project, if either its start date or finish date is determined for scheduling, the activity on the longest path will have zero TF. If the identified time period from start date to finish date is larger or less than the duration of the longest path, the activity on the longest path will have positive or negative TF. The longest path with positive TF means that the project has buffer time for executing the critical activities. On the contrary, the longest path with negative TF represents that the project does not have sufficient time for executing all activities on the longest path according to their scheduled dates.
For an activity, TF can be positive or negative. A positive TF for an activity indicates the amount of time within which the activity can be delayed without delaying the project finish date. A negative TF indicates the amount of time that must be saved so that the project finish date is not delayed. Negative float generally indicates that there is not enough time scheduled for the activity and is usually caused by constraint dates.
Maintaining positive floats for both the activity and project levels decreases the possibility of a project delay and increases the chance to complete the project on time or even ahead of the targeted completion date. Notably, when adopting PMSs for scheduling, a scheduler should use target dates for projects and activities carefully, particularly in the early stages of the planning process. The extensive use of target dates often has the effect of artificially distorting the patterns of float within a project, making analysis of the network more difficult.
Customizing Advanced Floats in PMSs
Most scheduling textbooks illustrate that an activity can have individual TF, FF, INTF and INDF. However, advanced floats (the floats excluding TF and FF) are ignored in most available PMSs. This fact implies that advanced floats are useless. Thus, software users no longer have the possibility to use those advanced floats by using available PMSs. A previous study has concluded that different float types can be used in different management scenarios. For example, FF plays an important role for subcontractors, whose own delays could cause a ripple effect on subcontractors further downstream; INTF measures how much delay can be absorbed within the entire project by delaying subcontractors [13].
Generally, advanced floats are proposed and derived based on the ADM approach. Most available PMSs no longer support the ADM approach due to complicated logical relationships that cannot easily be simulated by the ADM approach. However, whether using complicated logical relationships can improve the possibility of easily or successfully managing a project is an interesting question. Some PMSs provide customized fields for storing user-defined values. If the project manager and scheduler have knowledge and the capability to define the required float values by using available PMSs, it is possible for them to manage their projects based on more informative indicators. Furthermore, simply using TF is an acceptable choice for managing most projects. However, if the project’s logical relationships among activities are complex, it is necessary to employ other float types. This implies customizing advanced floats in PMSs is consequently necessary.
Managing Floats for Simplicity
There is no consensus on the ownership of project floats in the construction practice. Managing floats for simplicity or complexity appears to be in dilemma. If the floats ownership is not clearly described in a contract, the owner usually asks the contractor to arrange the floats for simplicity; however, the contractor always hides the floats for potential use, which makes managing floats complex because the reasonable floats belonging to the activities are difficult to identify.
Simplifying the use of floats should be a trend in PMSs although these systems provide comprehensive program options for the software user. The project stakeholders, particularly the owner and the contractor, should understand that completing a project on time or even ahead of the targeted dates should be a common project goal. Therefore, developing project schedules against a background of mutual trust and managing floats for simplicity would make project stakeholders focus on project execution and complete projects with mutual benefits.
PROACTIVE STRATEGIES FOR MITIGATING FLOAT-RELATED PROBLEMS
According to the information collected from the literature and prior discussions, this study further discusses three unsolved issues and proposes accompanying proactive strategies for mitigating the challenging float-related problems.
Clarifying Float Ownership
The ownership of project floats had received much attention in the past [2, 5]. Based on the construction practice and CPM theory, all float ownership options can be common divided into three categories: the owner, the contractor and the project [38-40]. Notably, float ownership discussion focuses merely on TF, which must be regarded as “common property” among the activities in the longest chain [41]. If a delay appears to influence an activity with positive TF, a dispute about the right to consume the TF usually arises. The contractor considers TF as a time contingency to cope with unanticipated conditions; on the contrary, the owner considers it as his/her right to use the float. Using an approach to pre-allocate TF would be an alternative for solving expected disputes [38].
The use of float as a resource should be reserved for the party who loses or gains as a result of fluctuations in the project cost [2]. Floats should be used on the basis of fundamental fairness and equity [2]. Based on the acknowledged fact that TF is beneficial to both the owner and contractor, a previous study proposed an approach to manage TF as a commodity with explicit contract language, which provides the contractor with the right to administer TF and the obligation to disclose its value and trade it on demand [5]. How to develop a clear and acceptable approach for measuring float consumption and the related liability, particularly when concurrent delays occur, and how to adjust the already existing knowledge and treatment to be on a basis of fairness and equity would be a challenge for researchers and practice. However, for better schedule management, clarifying float ownership in advance is a fundamental of proactive strategies on mitigating challenging float-related problems.
Understanding Relationship between Float and Critical Activity
In practice, an activity has one of the following criticality situations: (1) not critical; (2) critical-TF less than or equal to zero or a predefined value; (3) most critical-TF equal to the smallest value for the entire project; and (4) controlling critical-has float due to its own calendar but causes a successor to be critical [35]. It is clear that the so-called critical activity does not have a fixed float value when complex scheduling options are used. Therefore, a critical activity should be clearly defined with explicit scheduling specifications and contract language based on the project level. By using strong and clear scheduling specifications, the owner can help ensure that the contractor’s schedule realistically reflects the project requirements, the contractor’s approach to the work, and the status of the project [42]. Notably, most owners have no strong background knowledge to develop scheduling specifications.
To setup a transparent and manageable project schedule, many construction-related government agencies in the US, for example, the Departments of Transportation (DOTs) in the states of New Jersey, Virginia, and Texas, have developed different scheduling guidelines, checklists, specifications and best practices for the officer, the designer/engineer and the contractor to prepare to review and to update construction schedules [43-45]. It is quite frustrating that there are no clear specifications from the above-mentioned documents to define the relationship between float and critical activity. It could be an important research topic in the future.
For achieving better schedule management, to understand the relationship between float and critical activity in employed PMS and then to develop clear scheduling guideline would be another fundamental of proactive strategies on mitigating challenging float-related problems.
Managing Hidden Floats
When the start-to-start and finish-to-finish logical relationships are used in developing project schedules, the pitfall of hidden float (the float value is hidden and does not appear in TF or FF in employed PMS) usually appears in developed schedules. Traditionally, when an activity is defined as critical, any delay in this critical activity will delay the project completion date. Compared to traditional critical activity (both start and finish time are critical), a partial-critical activity is defined as that either the starting time or finish time is critical for the activity. For example, if an activity only has a start-to-start relationship to its successor and is on a critical path, then only its start time is critical. In such a situation, the activity usually has a hidden float. Therefore, it is necessary to manage the hidden float when complex project schedule is developed.
Several PMSs exhibit the hidden float in the format of a finish float. To manage the liability of schedule delay correctly, suitable float controlling is required. Managing a critical activity merely based on the criterion of critical/non-critical will lead to wrong information for decision making if complex logical relationships exist. It is suggested that avoiding employing start-to-start and finish-to-finish relationships is a basic principle in developing project schedules; otherwise, the scheduler should manage the start and finish float independently while using start-to-start and finish-to-finish relationships. How to develop a clear specification for detecting and managing hidden floats is an essential issue when complex logical relationships are used. There is no doubt that managing hidden floats aggressively is another fundamental of proactive strategies on mitigating float-related problems.
CONCLUSION
The CPM has been broadly used in managing construction project schedules in the past six decades. It is clear that only total float (TF) is widely used in schedule control and delay analysis. As the complexity of activity relationships and constraints in construction projects increased, simply managing the obtained float values usually results in more difficult schedule control and, consequently in incorrect decision making due to non-realistic float values. For providing comprehensive understanding of float in schedule management, this study thoroughly reviewed various float types, and discussed five managerial essentials consisting of representing flawless float values, managing correct floats in PMSs, maintaining positive floats, customizing advanced floats in PMSs, and managing floats for simplicity for better schedule management. Moreover, this study also discussed three proactive strategies consisting of clarifying float ownership, understanding relationship between float and critical activity, and managing hidden floats on mitigating challenging float-related problems based on the perspective of managing schedules by controlling floats.
Schedule management had received considerable attention, but the problems of float management still exist when managing complex construction projects. It is known that complicated logical relationships usually appear in construction projects. Therefore, the construction industry requires extra attention and proactive managerial actions to schedule float in better schedule management. The unsolved issues in proactive strategies on mitigating challenging float-related problems identified in this study provide a basis for further research. It is also suggested that using a demonstration case to illustrate the characteristics and differences for all discussed floats provides another comprehensive assessment but helpful one.
CONFLICT OF INTEREST
The author confirms that this article content has no conflict of interest.
ACKNOWLEDGEMENTS
The author would like to thank the National Science Council, Taiwan, ROC, for financially supporting this research under Contract No. NSC 102-2221-E-008-048-MY3.


